
Rapidity divergences arising in loop integrals are regulated by “tilted Wilson lines” prescription. So this spring constant then is the maximum torque divided by the distance from the pivot to the attachment point of the spring squared times sin of the angle that the loop sweeps multiplied by sin of the angle between the spring force and the lever arm and we have 8.83573 times 10 to the minus 7 newton meters from part (a) divided by 1.00 centimeter written as 1.00 times 10 to the minus 2 meters squared times sin 60 times sin 30 and that gives a spring constant of 1.59 times 10 to the minus 2 newtons per meter.The computation of one-loop corrections to Reggeon-Particle-Particle effective vertices with two scales of virtuality is considered in the framework of gauge-invariant effective field theory for Multi-Regge processes in QCD. So the torque then is the force that we found here times the lever arm times sin of the angle α here between the force and the lever arm and then we can plug in for F here- klsin Θ- and we end up with torque then is k times l squared times sin Θ times sin α and we'll solve for k by dividing both sides by l squared sin Θsin α.
#Loop the loop physics calculator full
And then we can talk about the torque, which we know from part (a) at a maximum- we have calculated what that will be- and at this full scale reading that's when you are gonna have the maximum torque because it carries 50 microamps at that point and we are told that that gives a full scale reading. So the force due to Hooke's law is the spring constant times the amount of stretching and the amount of stretching divided by the distance between the pivot and the attachment point l is sin of Θ and we can solve for Δ x by multiplying both sides by l here and so Δ x then is l times sin Θ we can plug that in to the formula for the force.
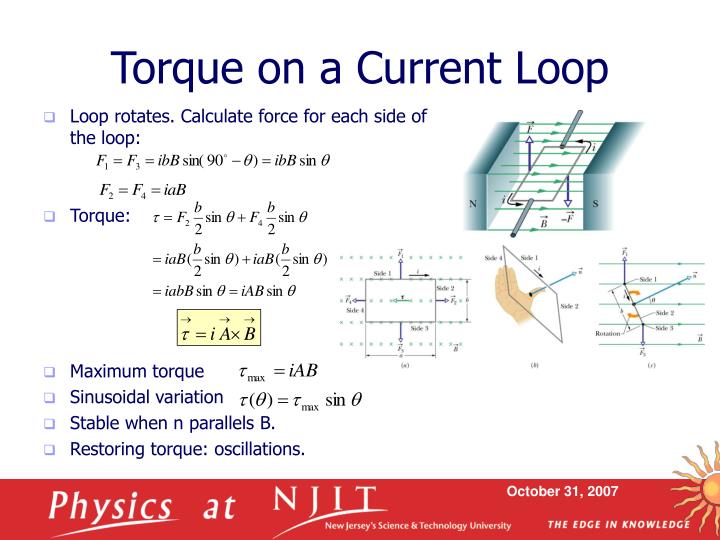
This angle is α and so this angle is important because the force due to the spring is going to have this angle with respect to the lever arm and so that's going to affect the torque created by the force and so the force is going to be directed this way due to the spring and we'll go force times the lever arm times sin of α to find the torque that it creates and α is 90 minus Θ and that is 30 degrees, 90 minus 60. So that's the assumption here 90 degrees there. So we are told the angle through which the loop moves, which is 60 degrees so here's the loop here and the spring is attached some distance l from the pivot and this l we are given is 1.00 centimeter and we are given this angle Θ and we are going to assume that there's a right triangle here and that assumption is close enough, it assumes that this attachment point for the spring is really far away from the loop in comparison to the distance between the pivot and the point of attachment because if it was close then when the spring is stretched, there will be some angle like this but we are gonna assume that that's negligible and the spring is still essentially horizontal when it is stretched. So it's gonna stretch it this much- Δ x- and we need to figure out what spring constant is needed such that we have this Δ x when there's a full scale reading. So when it does that, it's gonna stretch the spring that's holding the loop in its neutral position when there's no current. the scale would be like this now it's reading 50.0 microamps at full scale. So this loop will pivot so that it later on is like this and the needle is pointing down I didn't copy this scale there but you get the idea hopefully. Now in part (b), we are told that this coil is going to be used in a galvanometer that reads 50 amps at its maximum so there could be some indicator needle that is observed and it's going to sweep across this scale starting from zero going up to a maximum of 50 amps as this loop turns. So the area is π times radius squared since the loop is circular and we substitute that in for A and then we have 50 turns times 50 times 10 to the minus 6 amps of current times π times 1.50 times 10 to the minus 2 meters radius squared times 0.500 tesla, it works out to 8.84 times 10 to the minus 7 newton meters is the maximum torque. So the maximum torque happens when the plane of the loop is perpendicular to the field or you could say, the perpendicular of the loop is parallel to the field and that happens when you have the number of turns multiplied by the current times the area of the loop multiplied by field strength. We are going to find the maximum torque on a circular loop that has 50 turns in it and a radius of 1.50 centimeters carrying 50 microamps of current and a magnetic field that's 0.500 tesla.

This is College Physics Answers with Shaun Dychko.


 0 kommentar(er)
0 kommentar(er)
